Clik here: Animation on evolving grid
Clik here: Animation on evolving grid
Clik here: Animation on evolving grid
Clik here: Animation on evolving grid
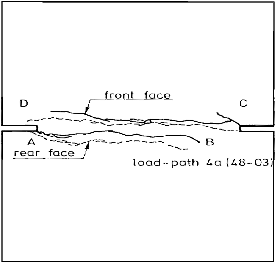
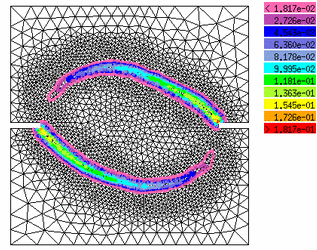
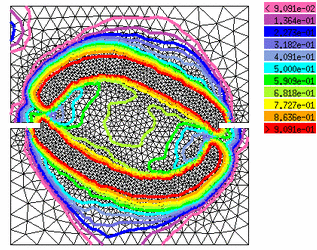
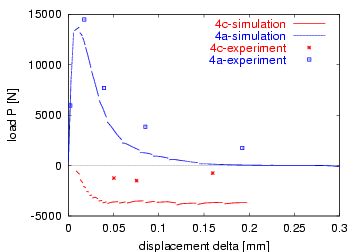
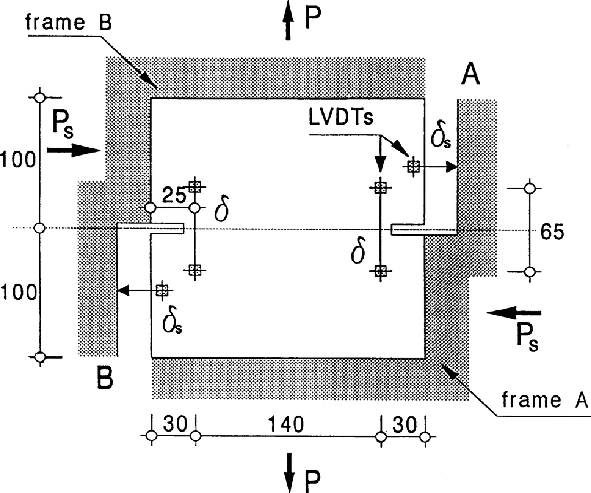 This double-edge-notched (DEN) specimen that was tested by Nooru-Mohamed
[1] is a nice example of damage propagation along a curved trajectory.
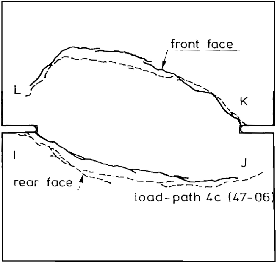
This double-edge-notched (DEN) specimen that was tested by Nooru-Mohamed
[1] is a nice example of damage propagation along a curved trajectory.| Test |
Experimental crack pattern |
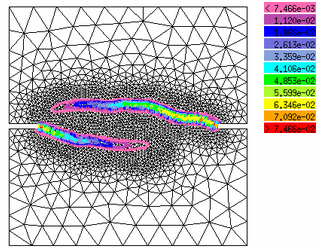
Principal strain |
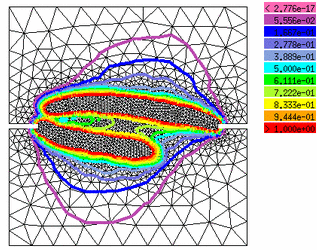
Damage indicator |
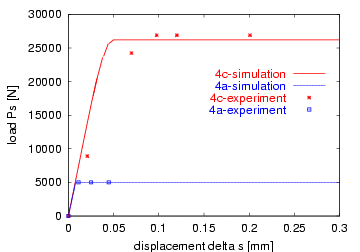
| 4a |

|

Clik here: Animation on evolving grid |

Clik here: Animation on evolving grid |
| 4c |

|

Clik here: Animation on evolving grid |

Clik here: Animation on evolving grid |
|
|
| This page is part of the OOFEM project documentation (www.oofem.org) (c) 2003 Borek Patzak, e-mail: info@oofem.org |

|